Maxwell-Boltzmann Distribution Law

Consider a system of N identical but distinguishable particles such as gas molecules at temperature T. Suppose the total volume of the system is V and total energy. Suppose n₁ molecules are present in the level with energy in the level with energy in the level with energy  and so on. However, whatever may be the type of distribution; the following two conditions must be satisfied:

                                     (Total number of molecules)
and                            (Total energy of the system)
Where n₁ is the number of molecules in the ith level with energy .

Many distributions are possible which satisfy the above two conditions. Now let us first see what the total number of such possible distributions is. The situation is similar to putting N numbered balls into n boxes such that n₁are in the fist box, n₂ in the second box and so on. Mathematically, the number of possible arrangement is given by
                                

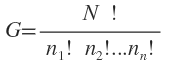
When applied to the system of N molecules, the number of possible distributions usually represented by W and is given by
                                 

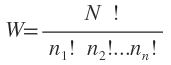
W is called the thermodynamic probability of the system because it is found that most of the thermodynamic properties are intimately related to the value of W ( e.g. S = k In W).

Now let us see ‘what is the most probable distribution’?

According to principles of statistical mechanics, the most probable distribution is one that makes W the maximum. This condition for maximum probability may be written mathematically in the form*
                              d = In W =0

Taking logarithm of both sides of equation we get
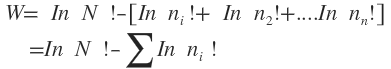         In
                   In

Applying Sterling’s formula, according to which
and                    

eqn. is simplified to the form
                           
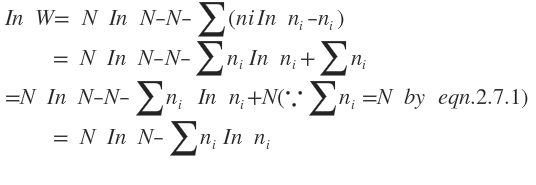
Differentiating both sides of this equating, we get
              
                                    {d(N In N) = 0,Because N is constant}
                                
(n_i In n_i has been differentiated as a product of two functions)
                        
But d In W = 0 (according to eqn.)
Eqn. gives
                         
Now as for the system under consideration   
  and                                              

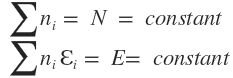
Therefore differentiation of these equations gives
and                         

Equations and (2.7.8), (2.7.9) and (2.7.10) represent the conditions which must be satisfied simultaneously for the most probable distribution.

Multiplying equations (2.7.9) and (2.7.10) by the undetermined constants and respectively and adding to eqn. (2.7.8) we get*
                                
                             
Putting another constant, eqn. (2.7.11) is further simplified to the form.
                            
Now within the restrictions imposed on the system for constant N and E values, the variation in (  ) are independent of each other and need not be zero, i.e., (   ) . Consequently, equation (2.7.12) will be satisfied only If the term in the summating is zero. Thus    
                                   
 
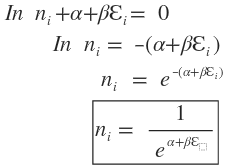
This expression is called Maxwell-Boltzmann distribution law

For more help in Maxwell-Boltzmann Distribution Law click the button below to submit your homework assignment