Orthorgonality
Orthorgonality Assignment Help | Orthorgonality Homework Help
Orthorgonality
This means that a particular wave function must be different from all other wave functions over the entire space. This is reasonable because if two particles had non-orthogonal wave function their description would not be unique as one wave function could be described in terms of the other. Therefore, the wave functions should be independent of one another . That is, orthogonal to one another.
The mathematical condition for orthogonally is that the product of the two wave functions, integrated over the entire space must be equal to zero This, if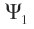 and
and 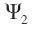 represent the two different acceptable wave functions of a system , then if,
represent the two different acceptable wave functions of a system , then if,

the two wave functions are said to be mutually orthogonal.
If, on the other hand, they obey the equations,
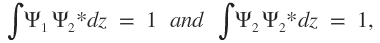
they are said to be normalized wave functions.
Wave functions which are both normalized and orthogonal are called orthonormal function .
For more help in Orthorgonality click the button below to submit your homework assignment
The mathematical condition for orthogonally is that the product of the two wave functions, integrated over the entire space must be equal to zero This, if

the two wave functions are said to be mutually orthogonal.
If, on the other hand, they obey the equations,
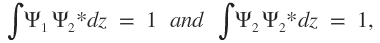
they are said to be normalized wave functions.
Wave functions which are both normalized and orthogonal are called orthonormal function .
For more help in Orthorgonality click the button below to submit your homework assignment