Current density

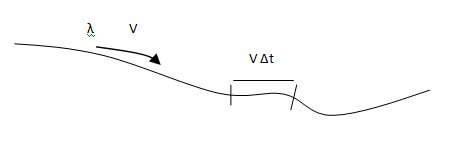
Consider a line charge λ travelling down a wire at speed v. A segment of length vΔt, carrying charge λvΔt, passes by point A in a time interval ∇t. The current in a wire is the charge per unit time passing a given point.
        I = dq/dt = λv Δt/Δt = λv                                … (1)
The magnetic force on a segment of current-carrying wire is
        Fmag = ∫(λdl)(v x B =  ∫ (I x B) dl                            … (2)

Since I and d I both point in the same direction,
        Fmag = ∫ I(d I x B)                                … (3)


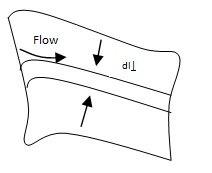
Surface Current Density. If, for some reason, the moving charges can be thought of as being constrained to flow on a surface, we can define a surface current density K. Its direction of flow of charge and its magnitude K is defined as equal to the current per unit length through a line lying in the surface and unit length through a line lying in the surface and oriented perpendicular to the flow. Consider a “ribbon” of infinitesimal width dl |, running parallel to the flow. Let the current in the ribbon be dI.

    . :    F = dI/dl |
Let the surface charge density be σ and its velocity be v.
The net line charge on the ribbon = σ dl| 
                dI = (σ dl|)v.
. :                K = σv                                 … (5)
The magnetic force on a surface current is
        F_mag = ∫(σ da)(v x B) = ∫(K x B)da                            … (6)


Volume Current Density (J). When the flow of charge is distributed throughout a three-dimensial region, we describe it by the volume current density J. Its direction is that of the direction of flow of charge and its magnitude J is given by the current per unit area through an area set perpendicular to the flow. Consider a “tube” of infinitesimal cross section da|, running parallel to the flow. Let the current in this tube be dI. Then
            J =  dI/da|                                 … (7)
Let the volume charge density be ρ,
Let the velocity of the moving charges be v. Then,
            J = ρv                                    … (8)
The magnetic force on a volume current is
        F_mag = ∫(ρ da)(v x B) = ∫(J x B) dτ                             … (9)


For more help in Current density please click the button below to submit your homework assignment