Earth Inductor

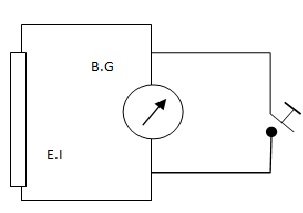
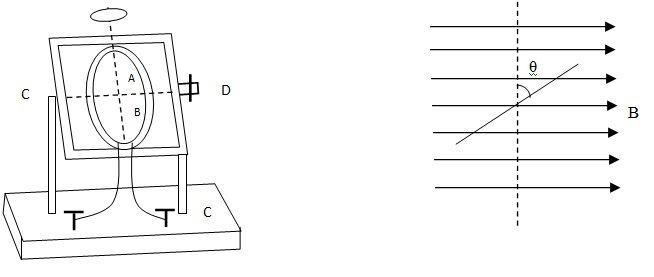
Earth inductor is an instrument used for the measurement of magnetic elements of earths. The purpose of an earth inductor is to generate an induced emf by virtue of the rotation of coil in earth’s magnetic field. Earth inductor is simply a circular coil consisting of a large number of turns of insulated Cu-wire wound over a wooden or ebonite frame. The coil is fitted in wooden frame. The coil can be rotated quickly through 180 about an axis passing through 180 about an axis passing through the centre of the coil and lying in its plane (i.e., about diameter AB). The frame itself can be rotated about an axis CD | to AB. Thus the axis of the rotation of the coil can be adjusted vertical or horizontal as desired. The ends of the coil are connected by means of flexible wired to two binding screws on the frame.

Theory. Consider a coil of N turns and cross-sectional area A placed in a uniform magnetic field of flux density B. At any instant t, the coil makes an angle to B.
The total magnetic flux passing through the coil = NBA cosθ
Hence the induced e.m.f. at this instant in the coil is given by
    E = - dΦ/dt = - d/dt (NBA cosθ) = - NBA d(cosθ)/dt
The charge, dq, flowing in the circuit in a time dt, is then
    dq = Idt = - NBA/R d(cosθ)                        ... (1)

Let q be the total charge passing through the B.G., when the coil is rotated through an angle 180 from θ₁ = 0 to θ₂ = π.

q= ∫dq= - NBA/R  ∫(θ₁=0)(θ₂=π)d(cosθ) = -NBA/R  [cosθ]0π= 2NBA/R

If θ₁ is the first throw of the B.G., due to this charge, then
        q = K θ₁ [1 + λ/2];
        2NBA /R = K θ₁ [1 + λ/2]                        … (2)

Where K is the ballistic reduction factor and λ is the logarithmic decrement.
The earth inductor can be used for the following purposes.

(a)    Determination of B_H.

To determine the value of the horizontal component of earth’s field (B_H), the earth inductor is connected in series with a B.G. through a rheostat and secondary coil S. The primary coil P is connected through a reversing-Key K to a battery, an ammeter A and rheostat and secondary coil S. The primary coil P is connected through a reversing-key K to a battery, an ammeter A and a rheostat. Initially the primary current in kept off. The coil is set with its axis of rotations vertical and the plane of the coil east-west, i.e., at right angles to the magnetic meridian. The coil is now rapidly rotated through 180 about the vertical axis. The corresponding first throw θ1 of the B.G. is noted.

Then             2NBA/R = Kθ₁ [1 + λ/2]
Where K and λ are the ballistic constant and logarithmic decrement.

In order to eliminate K and λ, the primary circuit is switched on and the current through it is adjusted to a suitable value. Let N₁ be the number of turn of the primary coil, l length of the primary coil and l the current flowing through it. Let N₂ be the number of turns of the secondary coil and a the area of each turn. Let R be the total resistance of the secondary circuit. If the current in the primary is suddenly reversed by means of the key K, the change in flux through the secondary is 2μ₀N₁N₂kla/l. Let q₂ be the charge passing through the B.G., now and θ2 the corresponding throw.
Then            q₂ = 2μ₀N₁N₂la/Rl = Kθ₂ [1 + λ/2]            … (2)

Dividing Eq. (1) by Eq. (2), we get
            NB_HAl/ μ₀N₁N₂la = θ₁/ θ₂
Or            BH = μ₀N₁N₂la / NAl (θ₁/ θ₂)            … (3)

(b)    Determination of B_v

The coil of the earth inductor is laid horizontally with the axis of rotation parallel to the magnetic meridian. The magnetic flux threading through the coil in this position, from fact 1 to face 2, is
NB_vA.
Where B_v is the vertical components of earth’s field. The horizontal component of earth’s field. The horizontal component does not pass through the coil at all.
The Procedure is the same as for determining B_H. If θ₁’be the throw of the ballistic galvanometer when the coil of the earth inductor is turned through 180, then
            Bv = μ₀N₁N₂la / NAl (θ₁/ θ₂)                    … (4)

(c)    Determination of Angle of Dip (Ñ„)

The earth inductor is connected directly to the B.G. The plane of the coil is first set vertical and at right angles to the magnetic meridian. The first throw θ1 is noted on rotation of the coil through 180. Then,
2NB_HA/R = Kθ₁ (1 + λ/2)                        … (5)

The coil is then set horizontal with axis of rotation parallel to the magnetic meridian and the procedure is repeated. Let θ₁’ be the first throw now. Then
            2NBvA/R = Kθ₁’ (1 + λ/2)                        … (6)
Dividing Eq. (6) by Eq. (5), B_v/B_H = θ₁’/ θ₁
Hence if Ñ„ be the angle of dip, then
        Tan Ñ„ = B_V/ B_H = θ₁’/ θ₁
Or        Ñ„ = tan^-1 (θ₁’/ θ₁)