Complex quantities, normally employed in ac circuit analysis, can be added and subtracted like coplanar vector. Such coplanar vectors which represent sinusoidally time varying. Quantities are called phasors.

Let the x-axis and y-axis projections of a phasor A be xA  is yA respectively. Then, its magnitude A is
        A = √(x₂A + y₂A)                                … (1)

The angle between the directions of phasor A and the direction of the positive x-axis is
        θ = tan^-1(yA/xA)                                    … (2)

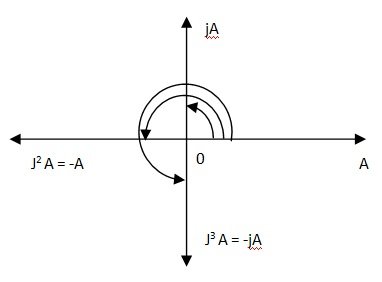
j is used to indicate the counter clockwise rotation of a phasor through 90o without changing its magnitude. It is assigned a value of √-1. When a given phasor A, the direction of which is along the x-axis, is multiplied by the operator j, a new phasor j A is obtained which will be 90o counter clock-wise from A i.e., along y-axis. If the operator j is applied now to the phasor jA, a new j2A is obtained which will be along –x-axis hand having same magnitude as of A. Thus J² A = -A.
Similarly,
        J³A = -jA.
        J⁴A = (√^-1)4 A=A.
Hence it is seen that the successive applications of the operator j to the phasor A produces successive 90^o steps of rotation of the phasor in the counter clockwise direction without in any way affecting the magnitude of the phasor.
Thus in Cartesian form, a phasor A can be written as
        A = a + jb,
Where a is the x-component and b the y-component of phasor A.

In polar form, the phasor can be written as
        A = A cos θ + jA sin θ = A (cos θ + j sin θ).
Here A cos θ is the x-component of the phasor and A sin θ the y-component. If the angle is measured in the clockwise direction from the x-axis, the phasor A can be written as
        A = A (cos θ – j sin θ).

Thus we see that cos θ + j sin θ is an operator which when applied with a phasor A, along the x-direction, rotates it through an angle θ from its initial position. Similarly the operator cos θ – j sin θ rotates the original phasor through an angle – θ.

In exponential form the phasor A can be written as
        A = Ae+ jθ = A (cos θ – j sin θ) = A+ θ

Addition of phasors. The sum of two phasors is a phasor which is defined in magnitude and phase position by he diagonal of parallelogram formed by the two phasors.
        A + B = C = B + A.

Subtraction of phasors. Phasors to be subtracted is rotated through 180^o and the addition is done by completing the parallelogram

Multiplication of phasors. The product of two phasors is a third phasor having magnitude equal to the algebraic sum of the individual phasor angles of these phasors with respect to the same reference axis.

        A = AejθA = A|θA and B = BejθB = B|θB
. :        AB = ABej(θA + θB)  = AB|θA + θB


Division of Phasors. When one phasor A is divided by another phasor B, it result in a phasor having magnitude A/B and the phase position with respect to the reference axis is the algebraic difference between the individual phase angles of these phasors with respect to the same reference axis.
Thus            A /B = C = AejθA /BejθB = A/B ej(θA – θB) = A/B|θA - θB

Raising of Power. A phasor may be raised to a given power n (an integer), by multiplying the phasor by itself n times.
        (A)n = An|nθA 

For more help in Phasor Algebra click the button below to submit your homework assignment.