Self-Inductance of a Toroidal Coil Rectangle Cross-Section

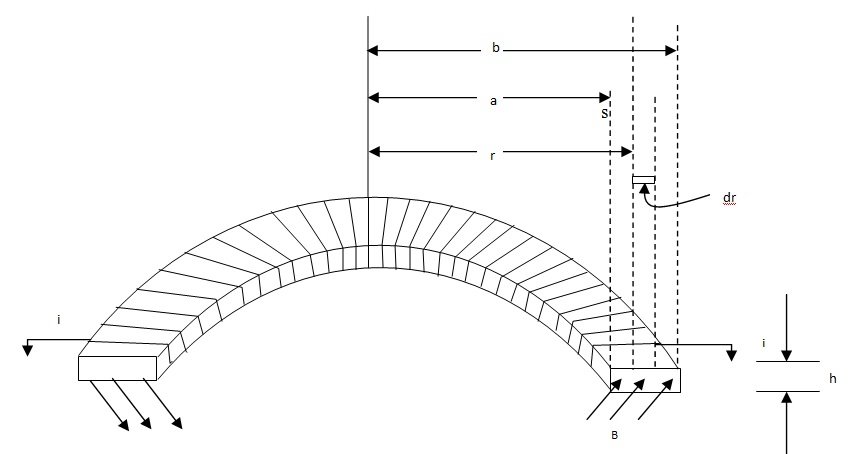
The section of a toroid having rectangular cross-section of thickness h. Let b and a b the external and internal radii of the toroid respectively. Let N be the number of turns uniformly distributed over its circumference.

The magnetic flux lines from concentric circles. They are restricted to the interior of toroid. They do not extend into the space beyond the windings. Consider a circular path of radius r concentric with the toroid. Let a current I flow through the winding. Let B be the magnitude of the magnetic induction produced at every point of the circular path due to the current.

Applying Ampere’s Law,
        φ B. dl = μ x current enclosed by the path
Since the magnetic field is tangential around the circular path of radius, r we have
        B. 2πr = μ (NI)
Or        B = μ (NI)/2πr

Here μ is the absolute permeability of the medium. Now, consider a rectangular strip of height h and width dr at a distance r from the centre. Area of this strip = hdr
    The magnetic flux linked with this strip is
        dφ = B. dA = B = hdr

Flux φ’ linked with one turn of the toroid is,
         φ’ = ∫ μ NI/ 2πr . hdr = μ NIh/ 2π ∫ DR/R = NIh/ 2π log_e b/a

Total flux φ linked with N turns of the toroid is
        φ = N φ’ = μ N2lh /2π log_e b/a

But according to definition of self-inductance,
        φ = LI
Therefore, the self-inductance L of the toroid is
        L = μN₂h /2π log_e b/a



For more help in Self-Inductance of a Toroidal Coil Rectangle Cross-Section please click the button below to submit your homework assignment.