Relation Between The Three Magnetic Vector B, H and M

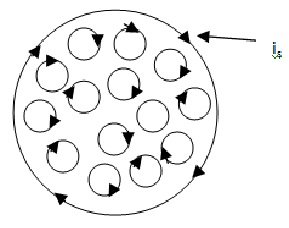
Consider a Rowland ring having a toroidal winding of winding of N turns around it. When a current i0 is sent through the winding, the ring is magnetized along its circumferential length. The current i0 is the real current which magnetizes the ring.

This magnetization arises due to the alignment of the elementary current-loops (magnetic dipoles) resulting from electronic motions in the materials. The small circles represent the current-loops. These internal tiny circular electron currents tend to cancel each other due to the fact that adjacent current are in opposite directions. As such there is no net current in the outer portions of the outer-most loops remain uncancelled. The numerous tiny localized surface currents can be replaced by a single closed current i5 along the surface. Such a current is called Amperian current.

Let A = area of cross and section and l = circumferential length of the ring. Then, volume of the ring = IA.
The ring behaves like a large dipole of magnetic movements isA.

Magnetization = M = magnetic moment per unit volume = i_NA/lA = iN/l .

The magnetization M, therefore is the surface current per unit lengths of the ring. This is commonly called magnetization currents.

Now, the magnetic induction B with in material of the ring arises due to the free current i0  in the winding, as well as due to the magnetization of the ring itself which can be described in terms of Amperian surface current.

. : = B = μ₀ (Ni₀/l + i_S/L) = μ₀ (Ni₀ /l +M)                     (.: i_s/L = M)

Here Ni0/l is the free current per unit and is/l is the Amperian surface current per unit length

. :     B/ μ₀ – M = Ni₀ /l                            … (1)

The quantity B/ μ₀ – M is called magnetizing field or magnetic field intensity H. i.e.,
        H = B/μ₀ – M
Or        B = μ₀ (H + M)                        … (2)
This is relation between the tree vectors B H and M points in the same direction as that of B or M and M. H points in the same direction as that of B or M. Its unit is Am-1

Eq. (1) can be written as H = Ni₀/l = ni₀

Where n is the number of turns per unit length. Thus the value of H depends only on the free current and is independent of the core material.
When no magnetic material is present in the core of the Rowland ring. i.e., there is vacuum in the core, M = 0. Therefore, Eq (2) becomes
            B₀ = μ₀ H                        … (3)
In vacuum, the magnetic field strength  H is related to the magnetic induction B0 by the above relation.

 When a magnetic material is placed in an external magnetic field, the specimen is magnetized by producing (or reorienting) magnetic dipoles in the specimen. This will produce additional field. Thus the resultant field B is greater than B0. In such a case, H is related to B by the relation,
            H = (B/μ₀) – M.