Uniform Constant Magnetic Field
Uniform Constant Magnetic Field Assignment Help | Uniform Constant Magnetic Field Homework Help
Motion of Charged Particle in Uniform Constant Magnetic Field
The magnetic force on a particle having a charge q moving with a velocity v in a magnetic field of magnetic induction B is given by
F = q(v x B) … (1)
|F| = q v B sin θ … (2)
(i) If the particle is at rest (v = 0), |F| = 0
(ii) If v and B are parallel or antiparallel, then |F| = 0
Let us now discuss two cases:
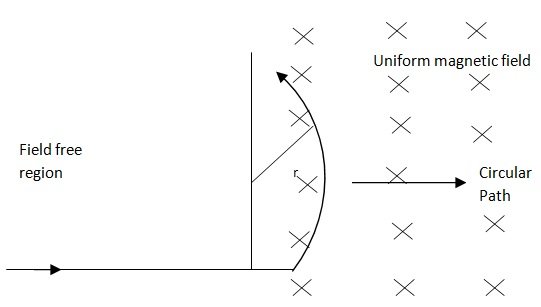
Case I. When the particle enters the magnetic field at right angles to the direction of the field.
Let v be the initial velocity of the particle normal to the direction of the magnetic field. The force due to magnetic field acting on the charged particle is perpendicular to both, the direction of motion of particle and the direction of the field. Hence, not work is done by the magnetic field on the charged particle. Thus the particle gains no kinetic energy and hence its velocity remains unchanged.
The magnetic force acting on the charged particle gives to centripetal force qvB. Hence, the path transverse by the particle will become a circle of radius r.
Here the magnetic field is shown perpendicular to the plane of the paper and directed into it.
Let m be the mass of the particle. Then,
F = qvB = mv2/r
The radius of the path is r = mv/qB … (4)
The radius r is called the gyro radius or cyclotron radius.
The angular velocity is ω = v/r = qB/m … (5)
The frequency is n = ω/2π = qB/2πm … (6)
This is called gyro frequency or cyclotron frequency of the charged particle.
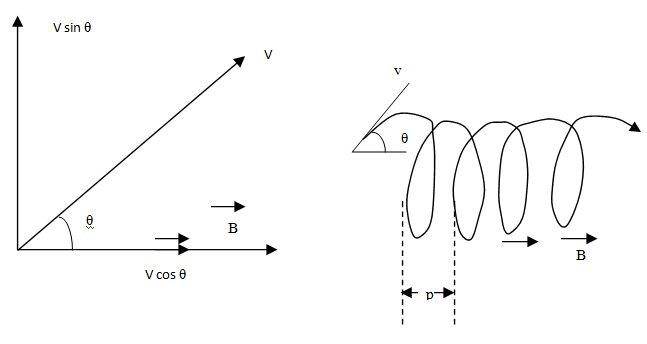
Case II. When the charged particle enters the magnetic field in any direction i.e., at an angle θ.
Let the charged particle of charge q and mass m enter the magnetic field making an angle θ with the direction of B. The velocity field making an angle θ with the direction of B. The velocity of the particle v may be resolved into components v cos θ and v sin θ, along and perpendicular to the direction of the applied field. The component v cos θ remains unaffected by the field. Hence the particle continues to move along the field with a speed of v cos θ. The velocity component v sin θ is in a plane perpendicular to the direction of the magnetic field. Hence the field exerts a force F on the particle making it to move along a circle in the plane with a constant speed v sin θ. The radius of the circular path is
R = mv sin θ / qB … (7)
Thus the charged particle possess two concurrent motions – one circular motion with a constant speed v sin θ in a plane perpendicular to the direction of magnetic field and another linear motion with constant speed v cos θ along the direction of the field. The resultant path of the charged particle is a helix.
The time taken by the particle for one revolution is given by
T = 2πr/v sin θ = 2π/v sin θ (mv sin θ/qB) = 2πm/qB … (8)
This distance travelled by the particle to the magnetic field in one revolution, i.e., in time T, is the pitch of th helix p.
p = (v cosθ) T = 2πmv cosθ /qB … (9)
For more help in Motion of Charged Particle in Uniform Constant Magnetic Field please click the button below to submit your homework assignment.
F = q(v x B) … (1)
|F| = q v B sin θ … (2)
(i) If the particle is at rest (v = 0), |F| = 0
(ii) If v and B are parallel or antiparallel, then |F| = 0
Let us now discuss two cases:
Case I. When the particle enters the magnetic field at right angles to the direction of the field.
Let v be the initial velocity of the particle normal to the direction of the magnetic field. The force due to magnetic field acting on the charged particle is perpendicular to both, the direction of motion of particle and the direction of the field. Hence, not work is done by the magnetic field on the charged particle. Thus the particle gains no kinetic energy and hence its velocity remains unchanged.
The magnetic force acting on the charged particle gives to centripetal force qvB. Hence, the path transverse by the particle will become a circle of radius r.
Here the magnetic field is shown perpendicular to the plane of the paper and directed into it.
Let m be the mass of the particle. Then,
F = qvB = mv2/r
The radius of the path is r = mv/qB … (4)
The radius r is called the gyro radius or cyclotron radius.
The angular velocity is ω = v/r = qB/m … (5)
The frequency is n = ω/2π = qB/2πm … (6)
This is called gyro frequency or cyclotron frequency of the charged particle.

Case II. When the charged particle enters the magnetic field in any direction i.e., at an angle θ.
Let the charged particle of charge q and mass m enter the magnetic field making an angle θ with the direction of B. The velocity field making an angle θ with the direction of B. The velocity of the particle v may be resolved into components v cos θ and v sin θ, along and perpendicular to the direction of the applied field. The component v cos θ remains unaffected by the field. Hence the particle continues to move along the field with a speed of v cos θ. The velocity component v sin θ is in a plane perpendicular to the direction of the magnetic field. Hence the field exerts a force F on the particle making it to move along a circle in the plane with a constant speed v sin θ. The radius of the circular path is
R = mv sin θ / qB … (7)
Thus the charged particle possess two concurrent motions – one circular motion with a constant speed v sin θ in a plane perpendicular to the direction of magnetic field and another linear motion with constant speed v cos θ along the direction of the field. The resultant path of the charged particle is a helix.
The time taken by the particle for one revolution is given by
T = 2πr/v sin θ = 2π/v sin θ (mv sin θ/qB) = 2πm/qB … (8)
This distance travelled by the particle to the magnetic field in one revolution, i.e., in time T, is the pitch of th helix p.
p = (v cosθ) T = 2πmv cosθ /qB … (9)

For more help in Motion of Charged Particle in Uniform Constant Magnetic Field please click the button below to submit your homework assignment.