When narrow gap between parallel plates or surfaces is filled by viscous fluid where the upper plate moves with uniform speed in the direction of flow and lower plate is fixed or stationary. Such a flow is called coquette flow.


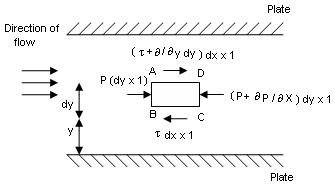
Consider two parallel fixed plates kept at a distance ‘t’ apart as shown in. A viscous fluid is flowing between these two plates form left to right. Consider a fluid element of length ‘dx’ and thickness ‘dy at a distance y from the lower fixed plate. If pressure intensity on face AB is ‘p’, then on CD will be    ( p + ∂p / ∂x dx ) and if shear stress on face BC is 'τ' , then on face AD will be  ( τ +  ∂τ / ∂y dy ).

Consider width of fluid element in direction perpendicular to paper is unity.

For steady and uniform flow, there is no acceleration and hence the resultant force in the direction of flow is zero.

p dy x 1 - ( p + ∂p / ∂x dx ) dy x 1 - τ dx x 1 + ( τ +  ∂τ / ∂y dy ) dx x 1 = 0

- ∂p / ∂x dx dy + ∂τ / ∂y dy dx = 0

∂p / ∂x  = ∂τ / ∂y

For more help in Couette flow click the button below to submit your homework assignment