Consider a uniform horizontal pipe having steady flow. Let fluid mass between section 1-1 and 2-2 as shown in.


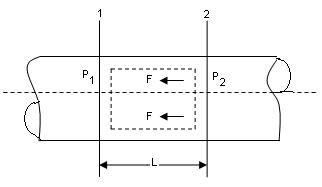
Let   A  =  Area of the pipe
        L   =  Length of pipe between
                 1-1 and 2-2

d = diameter of the pipe

P₁ and P₂ = pressure intensity at section 1-1 and section 2-2, respectively.
V1 and V2 = velocity at section 1-1 and section 2-2, respectively.

Applying Bernoulli’s equation between 1-1 and 2-2,

But Z₁ = Z₂ since the pipe is horizontal
       V₁ = V₂ since the pipe of uniform diameter,

P₁/ γ  P₂/ γ  + h_f

h_f  = P₁ - P₂ / γ

Where h_f = head loss due to friction

Experimentally it was found by Froude that the frictional resistance is given by,

Frientional resistance = Frictional resistance per unit wetted are a per unit velocity x Wetted
are x Velocity²

F = f ‘ x  π d L x V²

F = f ‘ P L V^₂

Since the velocity of flow is constant from section 1-1 to 2-2.

Net force in the direction of flow = 0

P₁ A – P₂ A – F = 0

F = (P₁ – P₂) A

Equating Equations

f P L V²  = (P₁ - P₂) A

P₁ - P₂ = f' P L V² / A

Now, equating Equations

γ. h_f  = f' P L V² / A

h_f  = f' / γ x P/A X LV²

Where hydraulic radius = P/A = wetted perimeter / Area = π d / πd² / 4 = 4/d

Putting f'/ρ= f/2 were f = coefficient of friction.

f'  f ρ/2

h_f  =  4 f L V² / 2g d

This is Darcy Weisbach equation for frictional loss
Sometimes Equation is written as,

h^f = f L V² / 2g d

Where f = friction factor = 4 x coefficient of friction

But  V = Q/A = 4Q / π d²

h_f = fL / 2g d (4q / πd²)² = 16 f L Q² / 2g π² d³

h_f = f L Q² / 12.1d³

For more help in Darcy-Weisbach Equation for Friction Loss click the button below to submit your homework assignment