Pascals Law
Pascals Law Assignment Help | Pascals Law Homework Help
Pascal’s Law
Pascal law states, “The intensity of pressure at a point in a fluid at rest is same in all direction.”
Pascal law: Consider an arbitrary fluid element of wedge shape in a fluid mass at rest as shown in. Let the width of the element be unity.
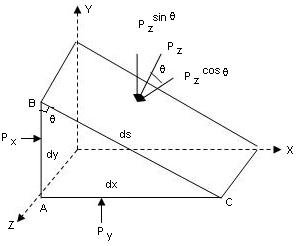
Let Px, Py and Pz be the pressures acting on the face AB, AC and BC respectively.
Let < ABC = ?
Forces acting on the element are
Force on the face AB = pressure in x-direction x Area of face AB
= Px (dy x 1)
Force on the face AC = pressure in y-direction x Area fo face AC
= Py (dy x 1)
Force on the face BC = pressure in z-direction x Area of face BC
= Pz (ds x1)
Weight of the element = V x γ = A x1x γ
= ½ x AC x AB x 1 x γ = dx.dy. γ / 2
Consider the triangle ABC as shown in
cos ? = AB/AC sin ? = AC/BC
C. cos ? = AB AC = BC. sin ?
ds. cos ? = dy dx = ds. sin ?
Resolve the force in x-direction
Px . dy x 1 - (Pz cos ?) ds x 1 = 0
P x dy = Pz . ds cos ? but ds cos ? = dy
Px. dy = Pz . dy
Px = Pz
Resolve the forces in y-direction
Py (dx x1) – P sin ? (ds x 1) -1/2 dx . dy . γ = 0
dx =dx . sin ? and also the element is very small and hence weight is negligible
Py . dx-Pz. dx = 0
Py = Pz
From equation (i) and (ii)
Px = Py = Pz
• Since the choice of fluid element was completely arbitrary, which means the pressure at any point is same in all direction.
• Pascal’s law states that , “The pressure or intensity of pressure at a point in a static fluid is same in all direction.”
• It is used for the construction of machines such as hydraulic press, hydraulic riveter etc. In which by the application of relatively small forces considerably larger forces are developed.
For more help in Pascal’s Law click the button below to submit your homework assignment
Pascal law: Consider an arbitrary fluid element of wedge shape in a fluid mass at rest as shown in. Let the width of the element be unity.
Let Px, Py and Pz be the pressures acting on the face AB, AC and BC respectively.
Let < ABC = ?
Forces acting on the element are
Force on the face AB = pressure in x-direction x Area of face AB
= Px (dy x 1)
Force on the face AC = pressure in y-direction x Area fo face AC
= Py (dy x 1)
Force on the face BC = pressure in z-direction x Area of face BC
= Pz (ds x1)
Weight of the element = V x γ = A x1x γ
= ½ x AC x AB x 1 x γ = dx.dy. γ / 2
Consider the triangle ABC as shown in
cos ? = AB/AC sin ? = AC/BC
C. cos ? = AB AC = BC. sin ?
ds. cos ? = dy dx = ds. sin ?
Resolve the force in x-direction
Px . dy x 1 - (Pz cos ?) ds x 1 = 0
P x dy = Pz . ds cos ? but ds cos ? = dy
Px. dy = Pz . dy
Px = Pz
Resolve the forces in y-direction
Py (dx x1) – P sin ? (ds x 1) -1/2 dx . dy . γ = 0
dx =dx . sin ? and also the element is very small and hence weight is negligible
Py . dx-Pz. dx = 0
Py = Pz
From equation (i) and (ii)
Px = Py = Pz
• Since the choice of fluid element was completely arbitrary, which means the pressure at any point is same in all direction.
• Pascal’s law states that , “The pressure or intensity of pressure at a point in a static fluid is same in all direction.”
• It is used for the construction of machines such as hydraulic press, hydraulic riveter etc. In which by the application of relatively small forces considerably larger forces are developed.
For more help in Pascal’s Law click the button below to submit your homework assignment